1 Introduction
Ever since its discovery, entanglement has become the most vital property of quantum information processing, playing a crucial role in quantum applications, including precise quantum metrology [1, 2], more powerful quantum computation [3], high security communication and networks [4, 5], and efficient simulation [6]. In this content, the generation and manipulation of complex entangled quantum states has been the major research direction for the purpose of bringing quantum technology superiority into full play [7]. One of the interesting classes of entangled states is the graph state, which can be represented by a graph, with vertices as qubits and edges as pair-wise interaction [8]. As a class of states with rich and unique entanglement properties, graphs have been applied to many fields of quantum information, leading to the research on the graph states in many different platforms [9,10,11].
Among the platforms for the physical realizations of quantum information, photon is an ideal information carrier for its low noise, fast transmission speed, various degrees of freedom and room-temperature operation [12]. For example, the first generation of graph state and demonstration of one-way computing was based on the bulk optics in 2005 [9], which is a milestone work of measurement-based quantum computing. As is mentioned above, the development of quantum technologies requires complex entangled states, which means the optical quantum systems become bigger and bigger. Under this circumstances, integrated optics, enabling localization and manipulation of photons at small scales, has become the ideal technology for the generation of entangled states [13], which has been demonstrated in various platforms: silicon [14], silica [15], lithium niobate [16], and gallium arsenide [17].
In this review, we summarizes state-of-the-art experimental progress and advances in the chip-based photonic graph states. Section 2 reviews the definition, theory and possible applications of the graph states, showing its necessity. In Section 3, we introduce the integrated optics as an ideal platform for generation and manipulation of complex entangled states, and introduce the progress of on-chip single-photon sources. In Section 4, we then focus on the experimental progress of the chip-based photonic graph states. Finally, in Section 5, we briefly discuss future development prospects and directions.
2 Quantum graph states: theory and applications
2.1 Definition of graph states
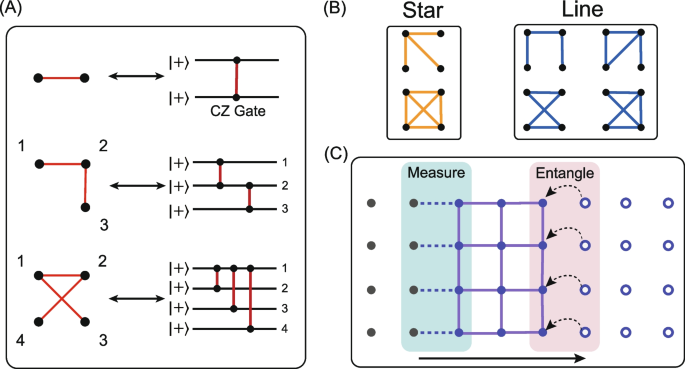
The definition of a graph state is mostly based on the mathematical definition for a graph. The illustration of a typical graph and its corresponding quantum state are shown in Fig. 1A. A graph G(V, E) is the combination of a set V of vertices and the edges E between them. Basically, the edge is not directional and can only connect two vertices. To construct a quantum state from a given graph, one can simply replace all vertices with qubits in \(\vert + \rangle = \tfrac{1}{2} ( \vert 0 \rangle + \vert 1 \rangle )\) and place a controlled-phase gate between any two of them that are connected by an edge in the graph. Controlled-phase gate, also named CZ gate, has the representation form of \(\text {CZ}=\text {I}-2\vert 11\rangle \langle 11 \vert\), flipping the phase only when both qubits are in state \(\vert 1\rangle\). Note here instead of giving a full expression of the graph state in some Hilbert space basis, we define it by construction and denote the quantum state by the corresponding graph. This is mainly because compared to the state expansion expression, the graph notation can show the entanglement between qubits more clearly and elegantly, which is closely related to the properties and applications of the quantum graph states.
Graph state: definition, classification, and applications. A Definition of graph state. As a special class of entangled state, graph state can be shown as a graph, where each vertex represents a qubit and the edge between vertices represents the entanglement relation of them. To prepare a given graph state, each qubit is first initialized into state \(\vert + \rangle\), then control-phase gate is performed on each pair of connected vertices. As the control-phase operator is indirectional, the edges in the graph are also indirectional. B Classification of four-qubit graph state. There are only two groups of graph state for the 4-qubit case, star-shaped and line-shaped. Graph states in the same group could be converted to each other by local operations only. C Dynamically growing of graph state used for universal quantum computing. The solid blue qubits in grid represent the current graph state, while the hollow blue qubits are waiting to be entangled into graph state and the gray ones are qubits that have been measured. The arrow indicates the direction of the quantum information flow
2.2 Properties and applications of graph states
As mentioned above, graph states have intriguing entanglement properties due to the CZ gates among qubits. For example, Fig. 1B shows the graph representation of four-qubit Greenberger-Horne-Zeilinger (GHZ) state (\(\text {GHZ}_4=\tfrac{1}{\sqrt{2}} \left( \vert 0000\rangle + \vert 1111\rangle \right)\) and linear cluster state. The different connection of the edge gives them different properties and applications, but both of them have been extensively studied for their importance and applications [18, 19]. Further, the complexity of the graph states grows quickly with the number of qubits involved. An important instance of such highly-entangled states is known as the cluster state [20], whose corresponding graph representation is a connected subset of a lattice. Cluster states can effectively simulate the Ising type interaction, and they found the basis of one-way computing. Since understanding entanglement of multi-qubit states is often the first step to harness them, the characterization of entanglement properties for graph states has been extensively studied [8, 21,22,23,24,25,26,27].
To realize quantum computation, it is necessary to have a set of universal gates upon which arbitrary unitary transformation can be built. For the optical platform where qubits are represented by photons, the realization of entangling gates have long been a problem since the interactions between photons are too weak. To go around this, people have come up with the scheme known as one-way quantum computing or measurement-based quantum computation (MBQC) [3]. An illustration of the idea is shown in Fig. 1C. Here, the entanglement between photons come from the initially prepared resource state, and one performs local measurements to effectively carry out all kinds of gates. Naturally entangled and scalable, graph states have shown great ability to play the role of resource states [3, 28, 29].
Another obstacle in the road of quantum computation is the noise from environment. Due to the fragility of the quantum states, noise could result in all kinds of errors like bit flip and phase flip. To overcome this problem, people have introduced the concept of quantum error correction, where the occurrence of an error can be detected and corrected by certain measurements on some specially designed quantum code state. It has been shown that the toric state and any stabilizer state can be transformed into a graph state by local Clifford operations [30, 31], which reveals the possibility to use graph states for error correction. Experiments of quantum error correction based on graph states has also been developed [32].
Apart from the above applications, graph states also fit themselves into several other fields. In quantum metrology, graph state can serve as a resource state that is robust against environmental noise [1]. They can also be used as states for quantum secret sharing [33, 34] because of their entanglement properties and elegant graph representation.
3 Integrated photonic quantum technologies
In the last 20 years, using the classical photonics tools and devices for quantum applications, integrated quantum photonics has become crucially important for scaling up and taking the quantum technology from laboratory to practical applications [13]. Since the first quantum application of integrated chip in 2008 [15], which demonstrated the controlled-NOT logic gate on silica-on-silicon optical waveguide circuits, the key devices for integrated photonic circuit in various platforms have been developed rapidly, including the high-quality single-photon sources to produce pure and indistinguishable photons with high efficiency based on nonlinear processes [35], and deterministic generation from quantum dots (QDs) [36], scalable and reconfigurable integrated quantum processors for the manipulation of the states [37], and the on-chip single-photon detectors to read out the quantum information [38].
3.1 Various integrated platforms
The integrated photonics generally has the advantages of scalablility, reconfigurablility, compact footprint, enhancing light-matter interaction, and high phase stability over the bulk optics. However, each platform has its own characteristics. For example, Silicon devices have rapidly grown and widely used for their tightly confinement of light and high-density integration [14]. III–V platforms and lithium niobate are able to integrate the active devices (light sources, switches and detectors), and to provide high nonlinearities, allowing for fast manipulations of single photons and fast feedforward [16, 39, 40]. Benefiting from mature micro-fabrication techniques and complementary metal-oxide-semiconductor (CMOS) compatibility, silicon has become an appealing candidate for generating, manipulating, and detecting large entangled states. Several major breakthroughs have been made over the years [31, 41,42,43].
3.2 Integrated single-photon sources
High-quality single-photon sources play a key role in the integrated photonics. Here, we want to review two types of integrated single-photon sources.
One is the probabilistic parametric photon-pair sources, known as heralded photon-pair sources. They are based on the spontaneous four-wave mixing (SFWM) or the spontaneous parametric down-conversion (SPDC) process [44] to produce the photon pairs following the conservations of momentum and energy, and have been demonstrated in different platform, including silicon [41], periodically poled lithium niobate [45], gallium arsenide [17], and silica [46]. There are many types of the parametric photon-pair source. First is the simple single-mode waveguides, always integrated into large arrays owing to their identical properties, where photons can be generated in a wide spectrum range. For example, in Ref. [41], an array of sixteen single-photon sources in silicon waveguides has been demonstrated to generate entanglement in high dimension. Since the parametric sources are probabilistic with low pair-generation rate, other techniques combining different modes of photon are needed to improve the efficiency, such as time [47], spatial [48], and wavelength [49] multiplexing techniques. However, broad-spectrum sources produce photons with low spectrum purity, which require filtering of the photon pairs with narrowband filters. This results in a trade-off between the purity and efficiency of the generated photon pairs. To solve this problem, a dual-mode pump-delayed excitation scheme is proposed, which utilizes inter-modal SFWM process to engineer phase matching and achieve spectral purity of 0.990 and intrinsic heralding efficiency of \(>90\%\) simultaneously. Using microresonators, including the microrings and microdisks, is another technique to improve the single-photon quality, which can reach \(95\%\) photon-number purity, \(50\%\) heralding efficiency and \(91\%\) indistinguishability and up to \(92\%\) spectral purity [50]. However, the locking between different resonators is still challenging.
The other approach is deterministic generation from QDs. Based on the semiconductor quantum dots including InAsP [51], GaAs [52], and InP [53], these single-photon sources are able to emit photons deterministically with high single-photon purity, efficiency and indistinguishability [36]. The main challenges are the requirement of hybrid integration techniques and the bad uniformity between different QDs. As for the solutions, breakthroughs have been made both in the integration of QD emitters with photonic waveguides using nanoscale positioning approaches [54], and the demultiplexing of the photons generated from a single QD [55], as well as realizing wavelength tunability to improve the uniformity [56, 57].
4 Experimental progress
4.1 On-chip graph states for MBQC
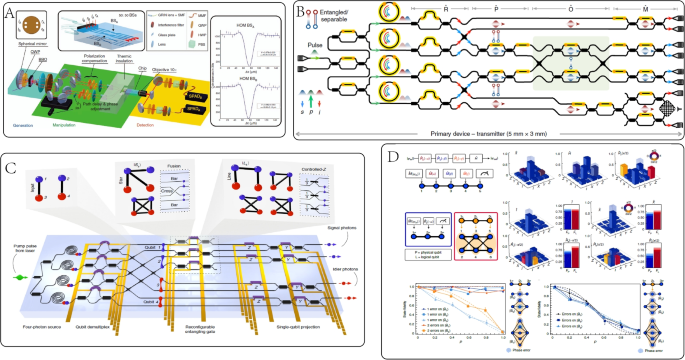
As is mentioned above, in the process of MBQC, different algorithms can be implemented by performing local measurements on the individual parties of the graph states. Experimental MBQC has already been firstly performed early in 2005 with bulk optics [9]. However, less experimental efforts in chip-based technologies have been performed, for the result of that graph states are multi-particle entangled states, but it is still difficult and challenging to generate multi-photon states on chip due to loss. In Ref. [58], encoding many qubits in different degrees of freedom (DOFs) of single photons, they realized four-qubit graph state on a SiO2 chip fabricated by femtosecond laser waveguide writing, which is the first one to manipulate graph state on chip. As shown in Fig. 2A, the system consists of a single-photon source, a manipulation stage, which includes the integrated photonic chip, and a detection stage. Here, using the scheme of hyper-entanglement, which means the entanglement in multiple DOFs, they encoded four-qubit information into pair-wise photons generated from spontaneous-parametric down-conversion. Built on the path and the polarization, the properties of four-qubit linear graph state were tested, and finally, they exploited the graph states to perform the Grover’s search algorithm, serving as the demo of MBQC.
However, as the first on-chip implementation of on-chip graph states and the algorithm according to one-way model, this experiment only integrated the manipulating circuits on chip, but lacked reconfigurability due to the material properties. The generation, programming, controlling and measurement of entangled states is at the heart of MBQC [37]. Integration of all these key functionalities and capabilities is the trend of the development.
4.2 On-chip genuine GHZ state
GHZ states are considered as an important class of graph states, also known as star-shaped graph states, and have a wide range of applications in quantum computing [59], quantum communication [60] and other fields. Because of its importance and its relative simplicity in experimental preparation, the GHZ state has been intensively studied in bulk optical quantum experiments. Since it’s first preparation, multi-photon GHZ state has been found to be unique in violation of local realism [61]. So far, GHZ state with 14-photon genuine entanglement,using single atom as photon source, has been reported [19].
In the field of integrated optical quantum chips, the number of entangled photons of GHZ state is limited to four, due to relatively large device loss and inadequate performance of single photon sources. In Ref. [50], the first on-chip four-photon GHZ state was reported, see Fig. 2B. An array of microresonator-enhanced single photon sources were used to increase spontanuous four wave mixing(SFWM) gain and suppress the noise outside of the sources, as well as to enhance the spectral purity of source. Three-photon and four-photon GHZ state were prepared by fusing two pairs of Bell states. Benefiting from the fact that the chip is highly reconfigurable, the operation of quantum gates and the projective measurements of quantum states were all performed on the chip. The fusion gate could be reprogrammed to realize other two-bit quantum operators, like Bell measurement, which was used to demonstrate quantum entanglement swapping and chip-to-chip quantum teleportation.
Besides qubit-based state, high-dimensional GHZ state is another hot topic in the field of quantum optics in recent years [62, 63]. With the advantages of highly identical single-photon source arrays and stable path encoding, integrated optical quantum chips provide a suitable platform for the preparation of multi-dimensional quantum states. Ref. [41] reported a multi-dimensional quantum experiment on large-scale silicon photonics chip, where a 2-photon 15-dimensional GHZ state was prepared. The entanglement was certified by quantum state tomography and dimension witness, showing high fidelity of the state and reconfigurability of the chip. Bell correlation in multi-dimension case was explored and stronger violation of LHV model was observed.
Graph state generation on photonic chip. A Hyperentanglement 4-qubit graph state. Two DoFs, polarization and path, are used to encode 4-qubit on a pair of photons. Hyperentanglement was generated in the blue part and manipulated in the orange part. On the chip, four path modes were processed by two beamsplitters. The Grover’s search algorithm based on the scheme of MBQC was demonstrated, showing the feasibility of using multiple DoFs on integrated photonic circuits to process quantum information. Adapted from Ref. [58]. B Four-photon GHZ state prepared on a silicon photonic chip. An array of microring resonantors were utilized to enhance photon number purity and joint spectral purity. A two-photon fusion operator was performed on two pairs of Bell states, resulting in a four-photon GHZ state. Entanglement witness was performed to ensure high fidelity of the prepared state. Adapted from Ref. [50]. C A reconfigurable 4-photon graph states on a silicon chip. A reconfigurable postselected entangling gate, which could be programmabled into fusion gate or control-Z gate, was integrated on the chip. On the same chip, both the star-shaped and line-shaped graph state could be prepared, consisting of the building block of measurement-based quantum computing protocol. Adapted from Ref. [43]. D Error-protected qubit based on graph state. Two pairs of 4-dimension Bell state were entangled into a 4-photon 4-dimension entangled state, then each qudit was encoded into two qubits, realizing an 8-qubit graph state. To protect against phase-flip error, each two physics qubit were encoded into a logical qubit, resulting in significant fidelity increasement compared to the situation without error correction. The result of measurement-based error-corrected state teleportation showed that multi-qubit repetition codes exhibited stronger error tolerance. What’s more, they show the success rate of phase-estimation algorithm increases from 62.5 to 95.8% without and with error protection. Adapted from Ref. [32]
4.3 On-chip programmable graph states
While different graph states correspond to different quantum computation tasks, only the generation of the specific graph state can not meet the need of future development, and the programmable generation of arbitrary graph states can accelerate their wide applications. Here, progress has been made in Ref. [43] based on a programmable silicon photonic chip. Four photons in two pairs are generated in superposition over four sources. As there are two classes of four-particle graph states under local unitary transformations, they generated all classes of four-photon graph state on chip, including the “star” state and the “line” state as shown in Fig. 2C. Moreover, with the high performance of silicon chip on the integrity and thermo-optic operation, this chip is able to implement a basic measurement-based protocol, and measure the entanglement properties of the four-photon graph states.
Taking advantages of the programmable circuits, this work integrates most of the key devices on chip, including bright four-photon source, manipulation circuit, and projective measurement part, which shows the high-performance control of quantum photonic chip, laying a foundation for the exploration of multi-photon entangled states and applications.
4.4 Hyper-entangled multi-photon graph states
A quantum device’s computational power is related to the Hilbert space accessible to it [4]. As for the optical version, the dimension of the Hilbert space is \(d^n\), where n is the photon number, and d is the dimension or mode number of each photon. As we introduced above, by increasing photon number n or the number of single-photon mode d(Dof.), some on-chip works are remarkable. Recently, combining multiple generations of entangled photon pairs and encoding of multiple qubits information into individual photons, four-photon eight-qubit graph states were generated on a mass-manufactured silicon photonic chip [32], which reaches the largest scale on chip-based graph state so far, as shown in Fig. 2D.
In this photonic chip, there are two maximally-entangled pairs of photons generated in the spiral sources and each photon encodes two-qubit information using the spatial mode mapping. What’s more, the stage of state processing and arbitrary projective measurements are also integrated on the same chip, showing the feasibility of high-dimensional encoding and multi-photon capability in integrated quantum photonics. Although the chip is not able to be programmed to generate all types of graph, they generated some typical ones and implemented the process of error-correction encoding and the MBQC phase estimation algorithm with physical and logical graphs (without and with error protection). This impressive resource-saving scheme provides inspiration for the future large-scale state generations and on-chip state dimension extension.
5 Outlook and conclusion
Entanglement plays a central role and forms the backbone in the applications of quantum technology, and graph state is an elegant class of the multi-particle entangled states, enabling profound applications in many quantum fields [8]. In this context, featuring scalable, controllable, robust, as well as low-cost, integrated photonics has become the trend of quantum technologies and exploits the opportunities for practical developments in the generation of quantum graph state [13]. So far, breakthroughs have been made on chip step by step, including the first hyper-entangled 4-qubit graph states in 2016 [58], the genuine four-photon GHZ state [50], the programmable four-photon graph state [43], and the reconfigurable four-photon 8-qubit graph state recently [32].
To date, most experimental work about chip-based graph has been implemented on the silicon-based devices, largely due to its mature fabrication, compact size and easy experimental manipulation. As is mentioned before, the major limitation of the qubit extension on chip is from the quality of quantum sources, including spectral purity and intrinsic efficiency, as well as photon loss during transmission. Fortunately, breakthroughs have been made in these two aspects. As for the high-quality single-photon source, referring to Section 3, improving techniques have been proposed and demonstrated, such as using the dual-mode pump-delayed scheme instead of the single-mode waveguides in silicon, or making full use of the different platforms, realizing the hybrid integration of QDs. For the relatively high transmission loss of silicon, the state-of-the-art silicon waveguides with low loss are improved to 0.08 dB/m [64].
However, individual platforms can not have the perfect performances across all domains. Due to the inescapable problems of the silicon, such as the two-photon absorption, loss, and negligible electro-optic coefficient, increasing the photon number, and realizing fast control for feed-forward still remain challenges on a single silicon chip [65]. A variety of other materials for integrated photonics have been developed, with their own features and advantages. For example, the silicon nitride provides negligible two-photon absorption and can create single photons within a broad transparent window [66]. Lithium niobate exhibit large electro-optic properties allowing for fast control and low loss [67]. InP has the ability of integrated the laser on chip and also allows for fast configuration [68]. It is worth expecting that the integration of different materials and devices monolithically on a single chip, known as hybrid or heterogeneous integration, is the dawn of a new era.
Besides, as for the entangled states, generalized from the graph states and allowing the presence of higher order correlations, hypergraph states as a new resources for quantum information have been proposed to apply in measurement-based protocols, quantum metrology applications [69,70,71]. In addition, the generation of high-dimension or d-level graph states provides increased quantum resources, a better noise tolerance, and also enables novel algorithms [72]. Beyond the graphs, going further to generate other significant but more complicated entangled states, making full use of the superiority of the integrated optics may also be next direction.